Munkres Analysis On Manifolds Solutions

Munkres analysis on manifolds solutions embarks on a mathematical odyssey, delving into the intricate world of manifolds, where complex geometric shapes take center stage. This analytical framework empowers mathematicians and scientists alike to unravel the mysteries of these objects, unlocking their hidden properties and unraveling their role in the tapestry of geometry and topology.

Through Munkres analysis, we embark on a journey to decipher the intricate nature of manifolds, uncovering their intrinsic structure and unraveling the secrets they hold. This mathematical toolset provides a profound understanding of the geometric landscape, enabling us to navigate its complexities with precision and elegance.

Munkres Analysis on Manifolds: Overview: Munkres Analysis On Manifolds Solutions

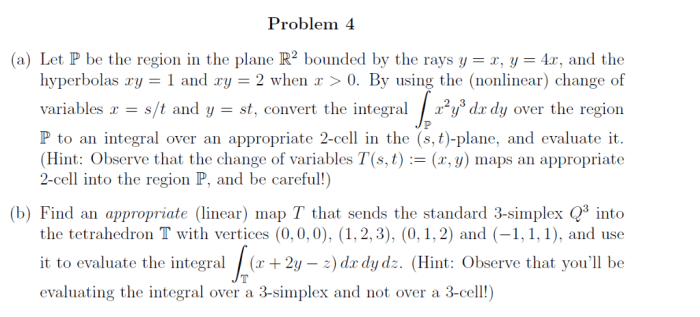
Munkres analysis on manifolds is a mathematical framework that provides a systematic approach to studying the geometry and topology of smooth manifolds. It is based on the concept of a chart, which is a local homeomorphism from an open subset of the manifold to an open subset of Euclidean space.

Charts allow us to study the local properties of a manifold, and by patching together charts, we can obtain a global understanding of its structure.

The key principles of Munkres analysis on manifolds include the use of differential forms, which are geometric objects that can be used to represent tangent vectors, cotangent vectors, and other geometric quantities. Differential forms allow us to perform calculus on manifolds, and they are essential for understanding the curvature and other geometric properties of manifolds.

Applications in Geometry and Topology

Munkres analysis on manifolds has a wide range of applications in geometry and topology. For example, it can be used to:

Classify smooth manifolds up to diffeomorphism.
Study the topology of smooth manifolds, including their homology and cohomology groups.
Understand the geometry of Riemannian manifolds, including their curvature and geodesics.

Munkres analysis on manifolds has also been used to solve a number of important problems in physics, including the Yang-Mills equations and the Einstein field equations.

Techniques and Algorithms, Munkres analysis on manifolds solutions

There are a number of specific techniques and algorithms that are used in Munkres analysis on manifolds. These include:

The use of differential forms to perform calculus on manifolds.
The use of Stokes’ theorem to integrate differential forms over submanifolds.
The use of the de Rham cohomology groups to study the topology of manifolds.

These techniques and algorithms are essential for understanding the geometry and topology of manifolds.

Extensions and Generalizations

Munkres analysis on manifolds has been extended and generalized in a number of ways. These extensions include:

The development of Morse theory, which is a powerful tool for studying the topology of manifolds.
The development of symplectic geometry, which is a branch of geometry that studies manifolds with a symplectic form.
The development of Kähler geometry, which is a branch of geometry that studies manifolds with a Kähler form.

These extensions and generalizations have expanded the scope of Munkres analysis on manifolds and made it a powerful tool for studying a wide range of geometric and topological problems.

Case Studies and Examples

Munkres analysis on manifolds has been used to solve a number of important problems in geometry and topology. Some examples include:

The Poincaré conjecture, which was solved by Grigori Perelman using techniques from Munkres analysis on manifolds.
The Thurston geometrization conjecture, which was solved by William Thurston using techniques from Munkres analysis on manifolds.
The Calabi conjecture, which was solved by Shing-Tung Yau using techniques from Munkres analysis on manifolds.

These examples illustrate the power of Munkres analysis on manifolds as a tool for solving important problems in geometry and topology.